Question

) The density of oil in a circular oil slick on the surface of the ocean at a distance of r meters from the center of the slick is given by δ(r)=401+r2 kilograms per square meter. Find the exact value of the mass of the oil slick if the slick extends from r=0 to r=5 meters.

Answer 1

Therefore the mass of the of the oil is 409.59 kg.

Explanation:

Let us consider a circular disk. The inner radius of the disk be r and the outer diameter of the disk be (r+Δr).

The area of the disk

=The area of the outer circle - The area of the inner circle

=
`\pi (r+\triangle r)^2- \pi r^2`

`=\pi [r^2+2r\triangle r+(\triangle r)^2-r^2]`

`=\pi [2r\triangle r+(\triangle r)^2]`

Since (Δr)² is very small, So it is ignorable.

∴
`A=2\pi r\triangle r`

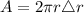
The density
`\delta (r)= (40)/(1+r^2)`

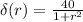
We know,

Mass= Area× density

`=(2r \pi \triangle r)((40)/(1+r^2)})`

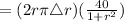
Total mass
`M=\sum_(i=1)^n (80r_i\pi )/(1+r^2)\triangle r_i`

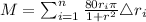
Therefore

`\sum_(i=1)^n (80r_i\pi )/(1+r^2)\triangle r_i=\int_0^5 (80r\pi )/(1+r^2)dr`

`=40\pi[ln(1+r^2)]_0^5`

`=40\pi [ln(1+5^2)-ln(1+0^2)]`

`=40\pi ln(26)`

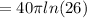
= 409.59 kg (approx)

Therefore the mass of the of the oil is 409.59 kg.