Final answer:
The area of a rectangle with a height of 4w^3 and a width of
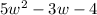 is given by the polynomial
is given by the polynomial
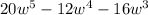 .
.
Step-by-step explanation:
To find the area of a rectangle, multiply the height by the width. Given a rectangle with a height of
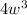 and width of
and width of
 the area is calculated as:
the area is calculated as:
Area = Height × Width =
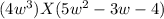
To compute the area, you need to perform the multiplication of these two polynomials:
Area =
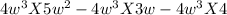
Area =
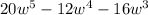
The area of the rectangle is
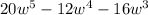 , which is a polynomial expression in terms of w.
, which is a polynomial expression in terms of w.