The age of Sari is 8 years.
The age of Justin is 9 years.
Step-by-step explanation:
Let x denote the age of Justin.
Let y denote the age of Sari.
Given that the product of their age is 72.
Thus, it can be written in equation as
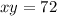
Since, the numbers x and y are consecutive integers and Justin is older, then, it can be written as,

Therefore, the two equations are
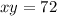 and
and

The ages of Sari and Justin:
The ages of Sari and Justin can be determined by solving the two equations.
Let us solve the equations using substitution method.
Substituting
 in the equation
in the equation
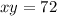 , we have;
, we have;
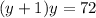
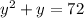
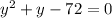
Factoring the equation, we get;
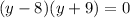
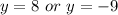
The value of y cannot be negative.
Thus,
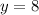 is the value of y.
is the value of y.
Substituting
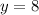 in the equation
in the equation
 , we have;
, we have;
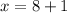

Thus, the value of x is 9.
Therefore, the age of Sari is 8 years and the age of Justin is 9 years.