Question

Can you please show work

Answer 1

The expression B.
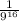`(1)/(9^(16) )` is equivalent to
`(9^(-2)) ^(8) .`

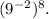
Explanation:

Step 1:

For the given number, -2 and 8 are both exponents.

According to the power rule,
`(a^(x)) ^(y) = a^(xy)`.

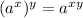Here a is 9, x is -2 and y is 8.

Substituting the values, we get
`(9^(-2)) ^(8) = 9^((-2)(8)) .`

`9^((-2)(8)) = 9^(-16) .`

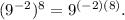
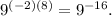
Step 2:

According to the negative exponent rule, we have
`a^(-z) = (1)/(a^(z) ) .`

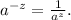
Here a is 9 whereas z is equal to 16.

So
`9^(-16) = (1)/(9^(16) ) .`

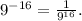
This is represented by option B.