The area of the given triangle is
 square inches.
square inches.
Explanation:
Step 1:
The area of a triangle is half the product of its base length and height.
The base is the lower end of the triangle and its length is called base length.
The length from the base to the highest point on the triangle is the height.
Step 2:
For the given triangle, the base extends for a length of (8x - 7) inches and the height is 6x inches.
The area of the given triangle
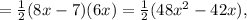
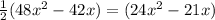 square inches.
square inches.
So the area of the given triangle is
 square inches.
square inches.