Question

At a highschool, 90% of the students take Physics and 35% of the students take both Physics and Statistics. What is the probability that a student that is taking Physics is also taking Statistics?

Answer 1

38.89% probability that a student that is taking Physics is also taking Statistics

Explanation:

We use the conditional probability formula to solve this question.

Suppose we have two events.

Event A and Event B.

The formula:

`P(B|A) = (P(A \cap B))/(P(A))`

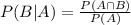
In which

P(B|A) is the probability of B happening, given that A has happened.

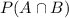`P(A \cap B)` is the probability of these two events happening.

P(A) is the probability of A happening.

In this problem, we have that:

Event A: taking physics

Event B: taking statistics.

90% of the students take Physics

This means that
`P(A) = 0.9`

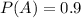
35% of the students take both Physics and Statistics.

This means that
`P(A \cap B) = 0.35`

What is the probability that a student that is taking Physics is also taking Statistics?

`P(B|A) = (P(A \cap B))/(P(A)) = (0.35)/(0.90) = 0.3889`

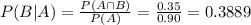
38.89% probability that a student that is taking Physics is also taking Statistics