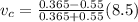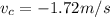Answer:
Part a)
Expression of speed of billiard ball is given as
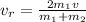
Part b)
Velocity of billiard ball is given as

Part c)
Expression for the speed of cue ball is given as
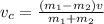
Part d)
Speed of cue ball is given as
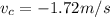
Step-by-step explanation:
Part a)
As we know that there is no external force on the system of two balls
so momentum is conserved here
so we have

also we know that the collision is elastic collision so we have
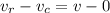
so we have
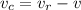
so we have

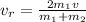
Part b)
Velocity of billiard ball is given as
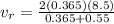

Part c)
Now the speed of cue ball is given as
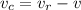
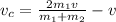
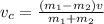
Part d)
Now by above formula the speed of the cue ball is given as