Question

An athletic field is a 60 yd60 yd​-by-120 yd120 yd ​rectangle, with a semicircle at each of the short sides. A running track 1010 yd wide surrounds the field. If the track is divided into eight lanes of equal​ width, with lane 1 being the​ inner-most and lane 8 being the​ outer-most lane, what is the distance around the track along the inside edge of each​ lane?

Answer 1

Distance of each track are:

D₁ = 428.5 yd

D₂ = 436.35 yd

D₃ = 444.20 yd

D₄ = 452.05 yd

D₅ = 459.91 yd

D₆ = 467.76 yd

D₇ = 475.61 yd

D₈ = 483.47 yd

Step-by-step explanation:

Given:

Track is divided into 8 lanes.

The length around each track is the two lengths of the rectangle plus the two lengths of the semi-circle with varying diameters.

Thus,

`D = 2(120) + 2. (1)/(2) \pi d\\\\D = 240 + \pi d`

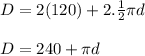
Starting from the innermost edge with a diameter of 60yd.

Each lane is 10/8 = 1.25yd

So, the diameter increases by 2(1.25) = 2.5 yd each lane going outward.

So, the distances are:

D₁ = 240 + π (60) → 428.5yd

D₂ = 240 + π(60 + 2.5) → 436.35 yd

D₃ = 240 + π(60 + 5) → 444.20 yd

D₄ = 240 + π(60 + 7.5) → 452.05 yd

D₅ = 240 + π(60 + 10) → 459.91 yd

D₆ = 24 + π(60 + 12.5) → 467.76 yd

D₇ = 240 + π(60 + 15) → 475.61 yd

D₈ = 240 + π(60 + 17.5) → 483.47 yd