Answer:
Therefore , every element of the function
 is a solution of the DE
is a solution of the DE
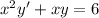 .
.
Explanation:
Given differential equation is
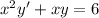
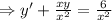 [ divided by x²]
[ divided by x²]
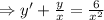
Here
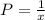 and
and
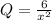
The integrating factor is =
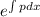
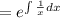

= x
Multiplying the integrating factor both sides of the ODE
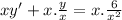

 [
[
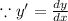 ]
]
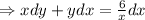
Integrating both sides
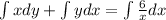
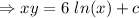 [ c is an arbitrary constant]
[ c is an arbitrary constant]
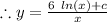 for x>0
for x>0
Therefore , every element of the function
 is a solution of the DE
is a solution of the DE
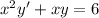 .
.