Question

The small washer is sliding down the cord OA. When it is at the midpoint, its speed is 28 m/s and its acceleration is 7 m/s 2 . Express the velocity and acceleration of the washer at this point in terms of its cylindrical components.

Answer 1

Velocity components

`V_r = -16.28 m/s`

`V_z = -22.8 m/s`

`V_q = 0 m/s`

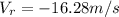
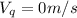
For Acceleration components;

`a_r = -4.07m/s^2`

`a_z = -5.70m/s^2`

`a_q = 0m/s^2`

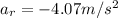
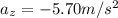
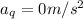
Step-by-step explanation:

We are given:

`Speed v_o = 28 m/s`

`Acceleration a_o= 7 m/s^2`

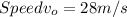
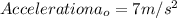
We first need to find the radial position r of washer in x-y plane.

Therefore

`r = √(300^2 + 400^2)`

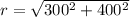
r = 500 mm

To find length along direction OA we have:

`L = √(500^2 + 700^2)</p><p>L = 860 mm`

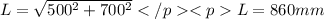
Therefore, the radial and vertical components of velocity will be given as:

`V_r = V_o*cos(Q)`

`V_z = V_o*sin(Q)`

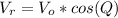
Where Q is the angle between OA and vector r.

Therefore,

`V_r = 28 * (r)/(L) = > 28 * (500)/(860)`

`V_r = -16.28 m/s`

•
`V_z = 28 * (700)/(860) = -22.8`

•
`V_q = 0 m/s`

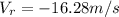
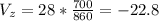
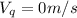
The radial and vertical components of acceleration will be:

`a_r = a_o*cos(Q)`

`a_z = a_o*sin(Q)`

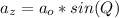
Therefore we have:

•
`a_r = 7* (500)/(860) = -4.07m/s^2`

•
`a_z = 7 * (700)/(860) = -5.70 m/s^2`

•
`a_q = 0 m/s^2`

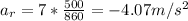
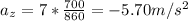
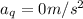
Note : image is missing, so I attached it