Answer:
The test statistic value is, z = 0.57.
The p-value of the test is 0.2843.
Step-by-step explanation:
A one-sided, single mean z-test can be performed to determine whether the mean caloric intake increased from 2403.7 kcal/day or not.
The hypothesis is defined as:
H₀: The mean caloric intake did not increase, i.e. μ = 2403.7.
Hₐ: The mean caloric intake did increase, i.e. μ > 2403.7.
The information provided is:

The z-statistic is:
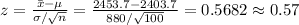
The test statistic value is, z = 0.57.
Compute the p-value of the test statistic as follows:
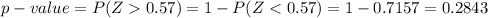
*Use a standard normal table.
The p-value of the test is 0.2843.