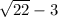Answer:
±
Explanation:
we would need to first separate the -13 to complete the square. To do that we divide 6 from the 6q by 2, which is 3. We then square the 3 to get positive 9. We separate the equation to become

since 9 - 22 is the same as -13
We can then reverse factor and get the equation to the form of:

Moving the -22 to the other side yields
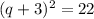
Taking the square root then isolating the q gives us the answers:
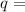 ±
±