Question

A restaurant sells about 330 sandwiches each day at a price of $6 each $.25 decrease in price. 15 more sandwiches are sold per day. How much should the restaurant charge to maximize daily revenue? Explain each step of your solution. What is the maximum daily revenue?

Answer 1

restaurant should charge $(6-0.25) = $5.75per sandwich to maximize daily revenue.

the revenue is $1983.75

Explanation:

to calculate current revenue= $6 x 330 = $1980

suppose x as the number of times the price to be dropped by $0.25

then find new price.. i.e

new price= $(6-0.25x)

and, new sell=330 +15x sandwiches

therefore, the new revenue would be= (6-0.25x)(330 +15x)

in order to maximize the current revenue, simplify the above equation and make it complete square using x

(6-0.25x)(330 +15x)

=1980-82.5x +90x -3.75
`x^(2)`

=1980 + 7.5x -3.75
`x^(2)`

=1980-3.75 (-2x+
`x^(2)`) ----> taking out common

now, to make a complete square lets add and subtract 1 inside the parentheses

=1980-3.75(-1+1-2x+
`x^(2)`)

=1980 +3.75 -3.75(
`x^(2)` -2x +1)

=1983.75 -3.75
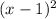`(x-1)^(2)`---->(1)

as
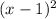`(x-1)^(2)` is positive always, minimize the other term in order to maximize the total revenue.

so the minimum possible value of
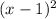`(x-1)^(2)` = 0

therefore, x=1

putting x in eq(1) the revenure becomes,

$(1983.75-0)=> $1983.75

therefore, restaurant should charge $(6-0.25) = $5.75per sandwich to maximize daily revenue.

the revenue is $1983.75