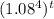Question

Which expressions are equivalent to 1.08^4t?

A.(1.08^t)^4

B.1.08^8t/1.08^2t

C.1.08^4*1.08^t

D.1.08^6t/1.08^2t

Answer 1

Therefore,

The Equivalent expression is option A,

`(1.08^(4))^(t)`

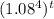
Explanation:

Given:

Expressions is

`1.08^(4t)`

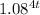
To Find:

Equivalent expression ?

Solution:

We have Law of indices

`(a^(x))^(y)=a^(x* y)\\(a^{\frac {x}{y}})^(z)=a^{(x* z)/(y)`

For option A

(1.08^t)^4

`(1.08^(4))^(t)=1.08^(4t)`

Hence option A is the Equivalent expression.

For option B

1.08^8t/1.08^2t

`(1.08^{(8t)/(1.08)})^(2t)=1.08^{(16t^(2))/(1.08)}=1.08^{14.81t^(2)}`

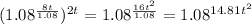
Which is not the Equivalent expression.

For option C

1.08^4*1.08^t

`(1.08^(4* 1.08))^(t)=1.08^(4.32t)`

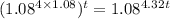
Which is not the Equivalent expression.

For option D

1.08^6t/1.08^2t

`(1.08^{(6t)/(1.08)})^(2t)=1.08^{11.11t^(2)}`

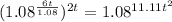
Which is not the Equivalent expression.

Therefore,

The Equivalent expression is option A,

`(1.08^(4))^(t)`