Question

The Company deposits $6,900 in an account that earns interest at an annual rate of 8%, compounded quarterly. The $6,900 plus earned interest must remain in the account 3 years before it can be withdrawn. How much money will be in the account at the end of 3 years?

Answer 1

$8750.87

Step-by-step explanation:

This is compound interest problem. The formula used to solve this would be:

`F=P(1+r)^t`

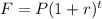
Where

F is the future value (what we want, after 3 years)

P is the initial value (given 6900)

r is the rate of interest per period

here, 8% per year, so 8/4 = 2% per period (since compounded per quarter)

t is the time (3 years and compounding per year so times of compounding is 3*4 = 12), so t = 12

Substituting, we get our answer:

`F=P(1+r)^t\\F=6900(1+0.02)^(12)\\F=6900(1.02)^(12)\\F=8750.87`

There will be about $8750.87 at the account at the end of 3 years!