Answer:
The towel bar should be placed at a distance of
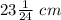 from each edge of the door.
from each edge of the door.
Explanation:
Given:
Length of the towel bar =
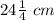
Now given length is in mixed fraction we will convert in fraction.
To Convert mixed fraction into fraction Multiply the whole number part by the fraction's denominator, then Add that to the numerator, then write the result on top of the denominator.
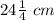 can be Rewritten as
can be Rewritten as
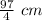
Length of the towel bar =
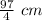
Length of the door =
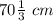
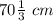 can be Rewritten as
can be Rewritten as
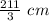
Length of the door =
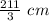
We need to find the distance bar should be place at from each edge of the door.
Solution:
Let the distance of bar from each edge of the door be 'x'.
So as we placed the towel bar in the center of the door it divides into two i.e. '2x'
Now we can say that;

Now we will take LCM to make the denominators common we get;
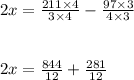
Now denominators are common so we will solve the numerators.
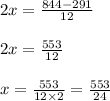
Or

Hence The towel bar should be placed at a distance of
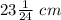 from each edge of the door.
from each edge of the door.