Answer:
3.75 feet.
Explanation:
Given:
Two people are standing next to each other on horizontal ground.
One is 5 feet tall, and the other is 6 feet tall.
The shadow of the person who is 6 feet tall is 3 feet shorter than twice the length of the shadow of the person who is 5 feet tall.
Question asked:
How long is the shorter person’s shadow?
Solution:
Let the shadow 5 feet person =

As given, shadow of 6 feet person is 3 feet shorter than twice the length of the shadow of 5 feet person.
Then, the shadow 6 feet person =

Now, as we know:
Ratio of the shadow of two objects are in proportion of their heights, so:-
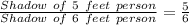
=
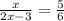
By cross multiplication:
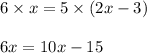
Subtracting both sides by
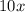
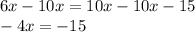
Adding both sides by minus
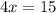
Dividing both sides by 4
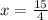
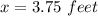
shadow 5 feet person =
 = 3.75 feet
= 3.75 feet
Therefore, shadow of the shorter person is 3.75 feet.