Question

UPPER AND LOWER BOUNDS

a book has a front and a back and 400 pages. the front and back cover are each 0.9 mm thick, measured to 1 decimal place. each page is 0.13 min thick, measured to two decimal places. calculate the minimum thickness of the book. some working must be shown

Answer 1

51.7mm

Explanation:

#Find the minimum bound of the back/front thickness:

-Given that it's rounded to one decimal point, the margin or error will be:

`ME=(1)/(2)*0.1\\\\=0.05`

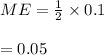
We subtract this ME from the front thickness to get the minimum thickness:

`M_t=0.9-0.05\\\\=0.85\ mm`

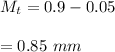
#Find the minimum bound of the pages:

-Given that it's rounded to one decimal point, the margin or error will be:

`ME=(1)/(2)*0.01\\\\=0.005`

We subtract this ME from the page thickness to get the minimum thickness:

`M_p=0.13-0.005\\\\=0.125\ mm`

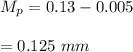
The minimum book thickness is thus calculated as:

`M_[bt}=2* M_t+400* M_p\\\\=0.85* 2+400* 0.125\\\\=51.7\ mm`

Hence, the minimum thickness of the book is 51.7 mm