Question

Solve the linear differential equation 2xy' + y = 2√x

Answer 1

Explanation:

General form of the linear differential equation can be written as:

`(dy)/(dx)+P(x)y=Q(x)`

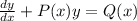
For this case, we can rewrite the equation as:

`(dy)/(dx)+(1)/(2x)y=(√(x))/(x)`

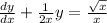
Here
`P(x) =(1)/(2x); Q(x)=(√(x))/(x)`

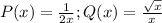
To find the solution (y(x)), we can use the integration factor method:

`Fy(x)=\int Q(x)Fdx+C \rightarrow F=e^{\int P(x)dx`

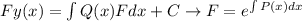
Then
`F=e^{\int (1)/(2x)dx}=e^\right=√(|x|)`

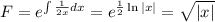
So, we can find:

`y√(|x|)=\int (√(x)√(|x|))/(x)dx+C`

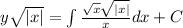
Suppose that
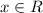`x\in \double R`, then
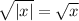`√(|x|)=√(x)` , and we find:

`y√(x)=x+C \rightarrow y(x)=√(x)+(C√(x))/(x)`

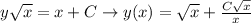
To check our solution is right or not, put your y(x) back to the ODE:

`y' = (1)/(2√(x))-\frac{C}{2\sqrt{x^(3)}}`

`2xy'=(x-C)/(√(x))`

`2xy'+y=(x-C)/(√(x))+√(x)+(C√(x))/(x)=2√(x)`

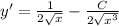
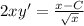
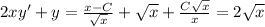
(it means your solution is right)