Question

The time it takes to deliver a pizza (from time of phone call to delivery at door) follows a normal distribution with a mean (µ) of 45 minutes and a standard deviation () of 5 minutes. If a pizza store’s policy is, "Orders delivered within one hour or they’re free!", what percentage of the store’s total delivery orders will be delivered to consumers with charge?

Answer 1

99.87% of the store’s total delivery orders will be delivered to consumers with charge

Explanation:

Problems of normally distributed samples are solved using the z-score formula.

In a set with mean
`\mu` and standard deviation
`\sigma`, the zscore of a measure X is given by:

`Z = (X - \mu)/(\sigma)`

The Z-score measures how many standard deviations the measure is from the mean. After finding the Z-score, we look at the z-score table and find the p-value associated with this z-score. This p-value is the probability that the value of the measure is smaller than X, that is, the percentile of X. Subtracting 1 by the pvalue, we get the probability that the value of the measure is greater than X.

In this problem, we have that:

`\mu = 45, \sigma = 5`

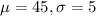
If a pizza store’s policy is, "Orders delivered within one hour or they’re free!", what percentage of the store’s total delivery orders will be delivered to consumers with charge?

Within one hour, which is 60 minutes. So this is the pvalue of Z when X = 60.

`Z = (X - \mu)/(\sigma)`

`Z = (60 - 45)/(5)`

`Z = 3`

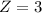
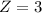`Z = 3` has a pvalue of 0.9987

99.87% of the store’s total delivery orders will be delivered to consumers with charge