Question

Is the line y=7x+2 perpendicular to the y=-1/7x+12 explain.

Answer 1

The line y = 7x + 2 perpendicular to the
`y=-(1)/(7) x+12`.

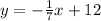Solution:

Equation of one line:

`y=7 x+2`

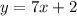
Slope of this line:

`m_1=7`

Equation of another line:

`$ y=-(1)/(7) x+12`

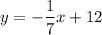
Slope of this line:

`$m_2=-(1)/(7)`

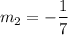
If two lines are perpendicular, then the product of their slope is -1.

`$m_1 * m_2=7 *(-1)/(7)`

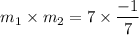
Both 7's get canceled, we get

`$m_1 * m_2=-1`

Therefore, product of slopes of the given lines are -1.

Hence, the line y = 7x + 2 perpendicular to the
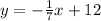`y=-(1)/(7) x+12`.