Question

Question18 (5pt)!!!!!!!!!!

Answer 1

sin θ =
`$(-√(33) )/(7)`

tan θ =
`$(-√(33))/(4)`

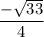
Explanation:

It is given that, cos θ =
`$(4)/(7)` then csc θ < 0, which implies that the θ is in the quadrant IV. Since cos θ is
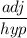`$(adj)/(hyp)` , we need to find the opposite side x.

Using the Pythogarus theorem, we can find the sin and the tan θ as,

7² = 4² + x²

x² = 7² - 4²

x² = 49 - 16

x² = 33

Taking sqrt on both sides, we will get,

x =
`√(33)`

Using the value of x, we can write the sine and tan ratio as,

sin θ =
`$(opp)/(hyp)` =
`$(-√(33) )/(7)`

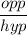tan θ =
`$(opp)/(adj)` =
`$(-√(33))/(4)`

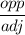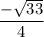
Thus we have obtained the values of sin θ and tan θ