Answer:
Largest possible total area of the four pens is

Explanation:
Assume width as x and length as y. Given that length of fencing is 950 feet which encloses rectangular area which is divided into four pens as shown in diagram ( Refer to attachment),
So perimeter of the rectangular area as per diagram is given as,
Perimeter = width + width + width + width + width + length+ length
Substituting the value,
 ….1
….1
Now area of rectangular diagram is given as follows,
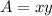 ….2
….2
Solving equation 1 for y, subtracting 2x from both sides,
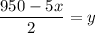
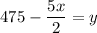
Substituting the value in equation 2,
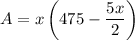
Simplifying
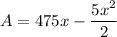
To find the largest possible area, differentiate A with respect to x,
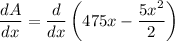
Applying sum rule of derivative,
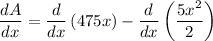
Applying constant multiple rule of derivative,
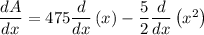
Applying power rule of derivative,
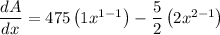
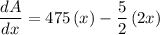
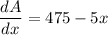
Now find the critical number by solving as follows,
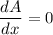
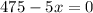
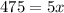
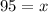
Since there is only one critical point, directly substitute the value of x into equation of A,
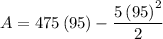
Simplifying,
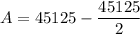
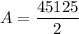
So, the largest possible area is
