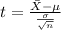Answer:
Null hypothesis :
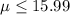
Alternative hypothesis:
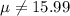
And the sample mean obtained was:

And the statitic to check this hypothesis is given by:
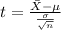
Explanation:
Previous concepts
A hypothesis is defined as "a speculation or theory based on insufficient evidence that lends itself to further testing and experimentation. With further testing, a hypothesis can usually be proven true or false".
The null hypothesis is defined as "a hypothesis that says there is no statistical significance between the two variables in the hypothesis. It is the hypothesis that the researcher is trying to disprove".
The alternative hypothesis is "just the inverse, or opposite, of the null hypothesis. It is the hypothesis that researcher is trying to prove".
Solution to the problem
On this case we want to proof is if the competitor sells the item for a mean price that differs from theirs. So this needs to be on the alternative hypothesis. And the complement of the alternative hypothesis would be on the null hypothesis.
Null hypothesis :
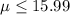
Alternative hypothesis:
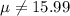
And the sample mean obtained was:

And the statitic to check this hypothesis is given by: