Question

You wish to buy a motor that will be used to lift a30-kg bundle of shingles from the ground to the roof of a house. The shingles are to have a1.5-m/s2 upward acceleration at the start of the lift. The very light pulley on the motor has a radius of 0.13m . Determine the minimum torque that the motor must be able to provide.

Answer 1

the minimum value of torque is 101.7 N-m.

Step-by-step explanation:

Given that,

Mass of bundle of shingles, m = 30 kg

Upward acceleration of the shingles,

The radius of the motor of the pulley, r = 0.3 m

Let T is the tension acting on the shingles when it is lifted up. It can be calculated as :

T-mg=ma

T=m(g+a)

`T=10* (9.8+1.5)\\T = 113 N`

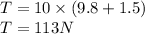
Let
`\tau` is the minimum torque that the motor must be able to provide. It is given by :

`\tau=r* T\tau=0.3* 339\tau=101.7\ N-m`

the minimum value of torque is
`101.7 N-m.`