Question

A sample of 1500 computer chips revealed that 32% of the chips do not fail in the first 1000 hours of their use. The company's promotional literature claimed that above 29% do not fail in the first 1000 hours of their use. Is there sufficient evidence at the 0.02 level to support the company's claim

Answer 1

Yes, we have sufficient evidence at the 0.02 level to support the company's claim.

Explanation:

We are given that a sample of 1500 computer chips revealed that 32% of the chips do not fail in the first 1000 hours of their use. The company's promotional literature claimed that above 29% do not fail in the first 1000 hours of their use.

Let Null Hypothesis,
`H_0` : p
`\leq` 0.29 {means that less than or equal to 29% do not fail in the first 1000 hours of their use}

Alternate Hypothesis,
`H_1` : p > 0.29 {means that more than 29% do not fail in the first 1000 hours of their use}

The test statics that will be used here is One-sample proportions test;

T.S. =
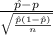`\frac{\hat p -p}{\sqrt{(\hat p(1-\hat p))/(n) } }` ~ N(0,1)

where,
`\hat p` = proportion of chips that do not fail in the first 1000 hours of their use = 32%

n = sample of chips = 1500

So, test statistics =
`\frac{0.32-0.29}{\sqrt{(0.32(1-0.32))/(1500) } }`

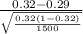
= 2.491

Now, at 0.02 level of significance the z table gives critical value of 2.054. Since our test statistics is more than the critical value of z so we have sufficient evidence to reject null hypothesis as it fall in the rejection region.

Therefore, we conclude that more than 29% do not fail in the first 1000 hours of their use which means we have sufficient evidence at the 0.02 level to support the company's claim.