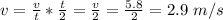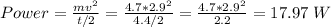Question

An initially stationary 4.7 kg object accelerates horizontally and uniformly to a speed of 5.8 m/s in 4.4 s. (a) In that 4.4 s interval, how much work is done on the object by the force accelerating it? What is the instantaneous power due to that force (b) at the end of the interval and (c) at the end of the first half of the interval?

Answer 1

(a) work done is 79.054 J

(b) power at the end of the interval is 35.93 W

(c) power at the end of the first half of the interval is 17.97 W

Step-by-step explanation:

Given;

mass of the object, m = 4.7 kg

final velocity, v = 5.8 m/s

time interval, t = 4.4 s

Part (a) work done on the object by the force accelerating it in 4.4 s interval

W = Fd = Δ KE

initial velocity, u = 0

W = ¹/₂mv²

W = ¹/₂ x 4.7 x (5.8)² = 79.054 J

Part (b) The instantaneous power due to that force at the end of the interval

Power = Fv

= mv/t x v

= mv²/t

= 4.7 x (5.8)² / 4.4

= 35.93 W

Part (c) power at first half of the interval

Power = Fv

velocity, v at half of t

v = u + at

v = at

t is half

v = a(t/2)

also a = v/t

`v = (v)/(t) *(t)/(2) = (v)/(2) = (5.8)/(2) = 2.9 \ m/s`

`Power= (mv^2)/(t/2) = (4.7*2.9^2)/(4.4/2) = (4.7*2.9^2)/(2.2) = 17.97 \ W`