Answer:
Explanation:
Given are four equations in three variables as
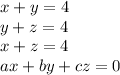
Let us take first three equations and solve
When we add we get 2(x+y+z) = 12
x+y+z = 6
Subtract from this equation the I equation to get z =2, similarly x =2, and y =2
If this is to be consistent with 4th equation we must have
2a+2b+2c =0
i.e. a +b+c =0
i.e. a =a, b =b and c = -a-b
Thre are infinite values for a,b,c to have x,y,z have solution as (2,2,2)
The system cannot have no solution or infinitely many solutions.