Question

Using traditional methods, it takes 11.5 hours to receive a basic driving license. A new license training method using Computer Aided Instruction (CAI) has been proposed. A researcher used the technique with 17 students and observed that they had a mean of 11.9 hours with a standard deviation of 1.7. A level of significance of 0.05 will be used to determine if the technique performs differently than the traditional method. Assume the population distribution is approximately normal. State the null and alternative hypotheses.

Answer 1

Null Hypothesis,
`H_0` :
`\mu` = 11.5 hours

Alternative Hypothesis,
`H_a` :
`\mu`
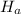`\\eq` 11.5 hours

Explanation:

We are given that using traditional methods, it takes 11.5 hours to receive a basic driving license. A new license training method using Computer Aided Instruction (CAI) has been proposed. A researcher used the technique with 17 students and observed that they had a mean of 11.9 hours with a standard deviation of 1.7.

We have to test the hypothesis that the technique performs differently than the traditional method or not.

Firstly, as we know that the testing is done always on the population parameter.

Let
`\mu` = mean time taken by the new license training method using Computer Aided Instruction (CAI) to receive a basic driving license.

SO, Null Hypothesis,
`H_0` :
`\mu` = 11.5 hours {means that the new license training method does not perform differently than the traditional method}

Alternate Hypothesis,
`H_a` :
`\mu`
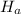`\\eq` 11.5 hours {means that the new license training method performs differently than the traditional method}

Also, the test statistics that will be used here is t-test statistics because we don't know about the population standard deviation.

We will calculate the test statistics and if this test statistics is more than the critical value of t at certain degree of freedom we will reject our null hypothesis or vice versa.