Question

In a experiment, the probability that event A occurs is 5/9, the probability that event B occurs is 5/7, and the probability that event A and B both occur is 3/8. What is the probability that A occurs given B occurs

Answer 1

answer: 52.5%

Good luck

Answer 2

52.5% probability that A occurs given B occurs

Explanation:

Suppose we have two events, A and B, the conditional probability formula is:

`P(A|B) = (P(A \cap B))/(P(B))`

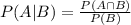
In which

P(A|B) is the probability of A happening given that B happened.

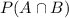`P(A \cap B)` is the probability of both A and B happening.

P(B) is the probability of B happening.

In this problem, we have that:

`P(A \cap B) = (3)/(8), P(B) = (5)/(7)`

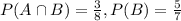
So

`P(A|B) = (P(A \cap B))/(P(B)) = ((3)/(8))/((5)/(7)) = 0.525`

52.5% probability that A occurs given B occurs