Question

Solve the equation.
4)x+ 5|- 6 = 6

Answer 1

x = -2, x = -8

Explanation:

I'm guessing the equation is 4|x+5| - 6 = 6. When there's an absolute value sign in the equation, we have two possible outcomes as |x| can be equal to x or -x. Thus, we have two values of x for which the equation will be true. Let's first split the equation into two:

`4(x+5)-6=6`

`4(-x-5)-6=6`

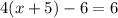
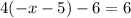
Now, solve both; first equation:

`4x+20-6=6`

`4x+14=6`

`4x=-8`

`x=-2`

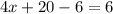
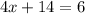
Second one:

`-4x-20-6=6`

`-4x=32`

`x = -8`

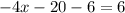
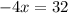
Thus, 4|x+5| - 6 = 6 has two real solutions: {x: x = -2, -8}