The area of the triangle XYZ is 29.1 square units
Step-by-step explanation:
Given that the measurements of the sides of the triangle XYZ are
 ,
,
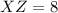 and
and
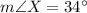
We need to determine the area of the triangle XYZ
Area of the triangle:
The area of the triangle can be determined using the formula,
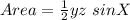
Substituting the values, we get,
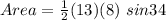
Simplifying, we get,
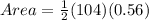
Multiplying the terms, we get,
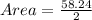
Dividing the terms, we have,
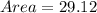
Rounding off to the nearest tenth, we get,
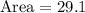
Thus, the area of the triangle XYZ is 29.1 square units.