Question

The Frooty Company is a family-owned business that produces fruit jam. The company has a grinding machine that has been in use for 3 years. On January 1, 2014, Frooty is considering the purchase of a new grinding machine. Frooty has two options: (1) continue using the old machine or (2) sell the old machine and purchase a new machine. The seller of the new machine isn’t offering a trade-in. The following information has been obtained:

Old Machine New Machine
The initial purchase cost of machines $150,000 $190,000
Useful life from acquisition date (years)
Expected annual cash operating costs
Variable cost per can of jam $0.25 $0.19
Total fixed costs $25,000 $24,000
Depreciation method for tax purposes MACRS, 7-year asset MACRS, 5-year asset
Estimated disposal value of machines
January 1, 2014 $68,000 $190,000
December 31, 2018 $12,000 $22,000
Expected cans of jam produced and sold each year
Frooty is subject to a 34% income tax rate. Assume that any gain or loss on the sale of machines is treated as an ordinary tax item and will affect the taxes paid by Frooty in the year in which it occurs. Frooty’s after-tax required rate of return is 12%. Assume all cash flows occur at year-end except for initial investment amounts.
Required:
1. A manager at Frooty asks you whether it should buy the new machine. To help in your analysis, calculate the following:
a. One-time after-tax cash effect of disposing of the old machine on January 1, 2014
b. The after-tax cash operating costs of keeping the old machine, of buying the new machine, and the difference between the two values (variable and fixed).
c. The total difference between the depreciation tax shield (DTS) resulting from keeping the old machine and buying the new machine.
d. The difference in after-tax cash flow from the terminal disposal of new machine and old machine
2. Use the net present value method to determine whether Frooty should use the old machine or acquire the new machine. Calculate the NPV of each option the way it was done in class. Check the NPV calculations using the NPV function in Excel.
3. How much more or less would the recurring after-tax cash operating savings of the new machine need to be for Frooty to be indifferent about whether to keep the old machine or buy the new machine? Assume that all other data about the investment do not change

Answer 1

Solution and Explanation:

Part 1 A

Original cost of old machine = 150000

Depreciation taken during first 3 years ((150000-20000)/8)*3 48750

Book value = 101250

Current disposal price = 68000

Loss on disposal = 33250

Tax rate = 34%

Tax savings from loss on current disposal of old machine = 11305

Total after tax cash effect of disposal = 68000 added 11305=79305

Part 1 B

Difference in recurring after-tax variable cash-operating savings, with 34% tax rate
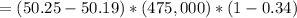`=(50.25-50.19) *(475,000) *(1-0.34)` = $18,810 (in favor of new machine)

Difference in after-tax fixed cost savings, with 34% tax rate
`=(\$ 25,000-\$ 24,000) *(1-0.34)`= $660 (in favor of new machine)

Part 1 c

Old machine New machine

Initial machine investment 150000 190000

Terminal disposal price at end of useful life 20000 25000

Depreciable base 130000 165000

Annual depreciation using straight-line (8-year life)16250

Annual depreciation using straight-line (5-year life) 33000

Annual income tax cash savings from difference in depreciation deduction =
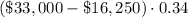`(\$ 33,000-\$ 16,250) \cdot 0.34` = $5,695 (in favor of new machine)

Part 1 D

Old machine New machine

Original cost 150000 190000

Total depreciation 130000 165000

Book value of machines on Dec. 31, 2018 20000 25000

Terminal disposal price of machines on Dec. 31, 2018 12000 22000

Loss on disposal of machines 8000 3000

Add tax savings on loss (34% of $8,000;

34% of $3,000 2720 1020

After-tax cash flow from terminal disposal of

machines ($12,000 + $2,720; $22,000 + $1,020) 14720 23020

Difference in after-tax cash flow from terminal disposal of machines = $23,020 minus $14,720 = $8,300 (in favor of new machine)

Part 2

2013 2014 2015 2016 2017 2018

Initial machine

investment (190000)

Current disposal

price of old machine 68000

Tax savings from

loss on disposal of

old machine 11305

Recurring after-tax cash-operating savings

Variable 18810 18810 18810 18810 18810

Fixed 660 660 660 660 660

Income tax cash savings

from difference in depreciation

deductions 5695 5695 5695 5695 5695

Additional after-tax cash flow

from terminal disposal

of new machine over old machine 8300

Net after-tax

cash flows (110695) 25165 25165 25165 25165 33465

Present value discount factors (at 12%) 1.000 0.893 0.797 0.712 0.636 0.567

Present value (110,695) 22472 20057 17917 16005 18975

Net present value (15269)

Considering NPV analysis, the Frooty Company should retain the old equipment because the NPV of the incremental cash flows from the new machine is negative.

Part 3

PVIF of $1 per year for 5 years discounted at 12% = 3.605. To make NPV = 0, Frooty needs to generate cash savings with NPV of $15,269.

$X multiply (3.605) = $15,269 (Let $X be the additional recurring after-tax cash operating savings required each year to make NPV = $0)

X = $15,269 divided by 3.605 = $4,235.51

Frooty must generate additional annual after-tax cash operating savings of $4,235.51.