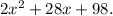Option B.
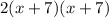 is the factorization of the polynomial
is the factorization of the polynomial
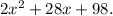
Explanation:
Step 1:
To determine which of the following options is the factor for the polynomial we can expand the given options and see which matches the given polynomial
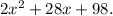
So we expand the four given options.
Step 2:
Option A.

Option B.
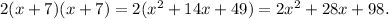
Option C.
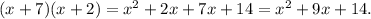
Option D.
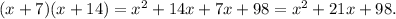
Only option B matches the given polynomial
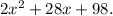 So option B is the factorization of the polynomial
So option B is the factorization of the polynomial