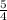The value of
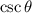 is
is
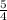
Step-by-step explanation:
Given that the function
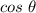 is
is

We need to determine the value of
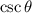
The value of Opposite side of the triangle:
The formula for
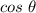 is given by
is given by
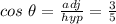
The value of opposite side can be determined using the formula,
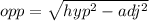
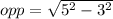
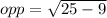
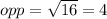
Thus, the value of opposite side is 4
The value of
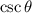 :
:
The formula for
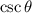 is given by
is given by
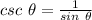
First, we shall determine the value of
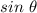
The formula for
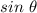 is given by
is given by
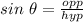
Substituting the values, we have,
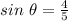
Thus, the value of
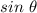 is
is
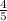
Substituting the values in the formula for
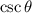 , we have,
, we have,
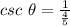

Hence, the value of
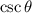 is
is