Answer:
12 milk chocolates.
Explanation:
-We know that probabilities add upto 1:

Let X be the total number of chocolates. We know that probability is the successful events divided by the total number of events:
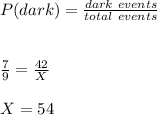
#We subtract the number of dark chocolates from X to get the number of milk chocolates:
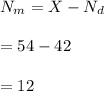
Hence, there must be 12 milk chocolates.