Write an equation in slope intercept form for the line with slope 3/2 and y intercept -1

Question

asked Jul 10, 2021 43.9k views

2 Answers

Tarmo · Answer 1 · 2021-07-11T22:27:53+0000

Answer:

y=3/2x+-1

Explanation:

the formula is y=mx+b

y is the total

m is the slope

x is what you're multiplying the slope by

b is the y intercept

Bits · Answer 2 · 2021-07-15T07:08:58+0000

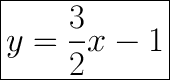
$\huge\boxed{y=(3)/(2)x-1}$

Start with the slope-intercept formula.

y=mx+b

In this formula,
is the slope and
is the y-intercept.

Substitute in the slope.

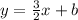
y=(3)/(2)x+b

Substitute in the y-intercept.

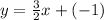
y=(3)/(2)x+(-1)

Adding a negative number is the same as subtracting the absolute value of that same number.

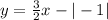
y=(3)/(2)x-|-1|

Find the absolute value.

$\large\boxed{y=(3)/(2)x-1}$