Question

Suppose (9, 14) and (3, −10) are the endpoints of a diameter of a circle. What is the equation of the circle?

Answer 1

The equation of circle is
`(x-6)^(2)` +
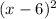`(y-2)^(2)` = 153

Explanation:

Given the endpoints of a diameter of a circle: (9,14) and (3,-10)

We know that the equation of a circle is

`(x-h)^(2)` +
`(y-k)^(2)` =
`r^(2)`

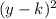where (x,y) is any point on the circle, (h,k) is center of the circle and r is radius of circle.

To find (h,k): the center is midpoint of diameter

Midpoint of diameter with end points (x1,y1) and (x2,y2) is given by

(
`(x1+x2)/(2)` ,
`(y1+y2)/(2)` )

(
`(9+3)/(2)` ,
`(14-10)/(2)` )

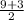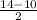(6,2)

Hence (h,k) is (6,2)

Substituting values of (h.k) and (x.y) as (6,2) and (9,14) respectively in equation of circle, we get

`(9-6)^(2)` +
`(14-2)^(2)` =
`r^(2)`

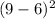`r^(2)` = 153

Substituting the values of (h,K) and
`r^(2)`, we get the equation of circle as

`(x-6)^(2)` +
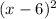`(y-2)^(2)` = 153

Hence the equation of circle is
`(x-6)^(2)` +
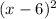`(y-2)^(2)` = 153