Answer:
Inverse of function g = {(1,a),(2,b),(3,2),(4,d)}
Explanation:
Hey there! :)
Please see an explanation below for better understanding to the answer - also let me know if you have any questions!
What is Inverse Function?
Inverse Function is a function that swaps domain and range.
What are domain and range?
Domain is the set of all x-values while Range is the set of all y-values, can be defined in coordinate point as (x,y).
Steps
If (x,y) is an original function then (y,x) will be an inverse function of original.
We are given the function g = {(a,1),(b,2),(2,3),(d,4)} - swap the domain and range.
Therefore, the inverse of function g is
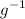 = {(1,a),(2,b),(3,2),(4,d)}
= {(1,a),(2,b),(3,2),(4,d)}