Answer:
The answers to the question are;
(a) The distance the box slides before stopping is 5.11 m.
(b) The coefficient of friction at the stopping point is 0.304 m
(c) The distance the box would have slid if the friction coefficient didn’t increase but instead had the constant value of 0.100 is 10.32 m
Step-by-step explanation:
Here, we note that
Initial velocity of box, v = 4.50 m/s
Final velocity of box, u = 0 m/s
Start friction of rough section μ₀ = 0.100
Max friction μ
 = 0.600
= 0.600
Distance of friction increase = 12.5 m
Therefore since μ varies with distance linearly, we have
The slope given by
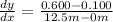 = 0.04
= 0.04
Therefore the equation is
f(μ) = Ax + B
and when x = 0, (The starting point) μ₀ = 0.100
Therefore B = 0.100 and A = The slope = 0.04
The equation is μ(x) = Ax + B = 0.04·x + 0.100
The work done is then found by summing the work done along the length of the rough path as follows
W =

Which gives W = -m·g·(0.02·x²+0.1·x)
Equating the work done to the change i kinetic energy, we have,
 =-m·g·(0.02·x²+0.1·x)
=-m·g·(0.02·x²+0.1·x)
From where we have
 ((4.50 m/s)²- (0 m/s)²) = 9.81×0.02·x² + 0.1·x = 0.1962·x² + 0.981·x
((4.50 m/s)²- (0 m/s)²) = 9.81×0.02·x² + 0.1·x = 0.1962·x² + 0.981·x
10.125 m²/s² = 0.1962·x² + 0.981·x
That is 0.1962·x² + 0.981·x- 10.125 m²/s² = 0
Dividing both sides by 0.1962, we get
x² + 5·x - 51.61 = 0
Factorizing, we have
(x+10.11)(x-5.11) = 0
Therefore x = -10.11 m/s or x = 5.11 m
Since we are working with positive values of motion, the proper solution is
x = 5.11 m.
(b) The coefficient of friction at the stopping point is given by;
Substituting the value of x into the equation for increasing friction, we get
μ(x) = 0.04·x + 0.100 → 0.04·5.11 + 0.100 = 0.304 m
Coefficient of friction at stopping point μ(x) = 0.304 m.
(c) With a constant frictional force, we have
F = -μ·m·g
Work done = Force × Distance = -μ·m·g·x =

Therefore
-μ·g·x =

-0.1 × 9.81×x =
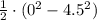
x =
 = 10.32 m
= 10.32 m
The distance the box will slide under constant friction is 10.32 m.