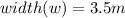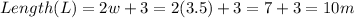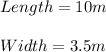Question

The area of a rectangle is 35 m² in the length of rectangle is 3 m more than twice the width find the dimensions of the rectangle

Answer 1

`Length=10 m\\\\Width=3.5 m`

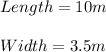
Explanation:

Area of the rectangle =
`35 m^2`

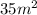
Let the width of the rectangle be 'w' meter

Length of the rectangle=
`(2w+3) meter`

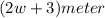
Area of the rectangle = length * Width

`35=(2w+3)(w)\\\\35=2w^2+3w\\\\2w^2+3w-35=0`

Making the factors of the equation:

`2w^2+10w-7w-35=0\\`

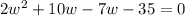
Taking common form the equation:

`2w(w+5)-7(w+5)=0\\\\(w+5)(2w-7)=0\\`

`(w+5)=0\\\\w=-5`

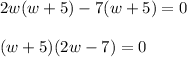
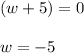
OR

`2w-7=0\\\\2w=7\\\\w=7/2\\\\w=3.5m\\\\`

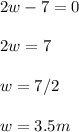
As, the width of the rectangle cannot be negative so the
`width(w)= 3.5 m`

`Length(L)=2w+3= 2(3.5)+3= 7+3=10 m`

`Length=10 m\\\\Width=3.5 m`