Question

Consider two projectiles that can be shot upward by spring guns. Object A is made of solid aluminum and has a mass of 50 grams. Object B is made of cast iron and has a mass of 200 grams. For this problem, you may take the gravitational field strength to be g = 10 N/kg.

(a) Objects A and B are both shot straight upward at the same time. Mass A is shot with a speed of 2 m/s and mass B with a speed of 1 m/s.
Which one hits the ground first?
(b) Find an equation that expresses how high a ball rises, if it is shot straight up with a speed v0, it has a mass m, and the gravitational field strength is g. Solve h= in terms of g, m, and v0.

Answer 1

(a) Object B hits the ground first.

(b)
`h = (V_0^2)/(2g)`

h = 0.2 meters (for object A)

h = 0.1 meters (for object B)

Step-by-step explanation:

(a) For this part, we simply need to use the equations of motion as follows:

`V^2 - U^2 = 2*a*s`

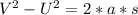
This will help us find the distance (s) both objects travel in total. The distance to the highest point will be:

V = 0

U = 2 m/s

`0^2-2^2=2*(-10)*s`

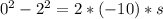
s = 0.2 meters (for object A)

for object B, we have:

V= 0

U = 1 m/s

`0^2-1^2=2*(-10)*s`

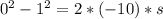
s = 0.1 meters (for object B)

The total distance traveled will be twice the distance to the highest point, as the objects have to come back to the ground the same amount of distance.

This means:

s = 0.4 (for object A)

s = 0.2 (for object B)

We can now use the following equation of motion to find the time:

`s=u*t+(1)/(2) (a*t^2)`

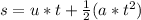
Plugging in the values of s (total distance) , u (initial speed) and a (acceleration) we get the following answers for each:

t = 0.1464 seconds (for object A)

t = 0.1236 seconds (for object B)

Object B hits the ground first.

(b) It is important to note here that mass does not have any affect on how high a ball rises. All that matters is the initial speed v0, and acceleration due to gravity. We may have the following equation for this purpose:

`V^2 - U^2 = 2*g*h`

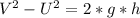
where U = V0

V = 0

g = acceleration due to gravity

and h = distance traveled

Rearranging, we get:

`h = (V_0^2)/(2g)`

Since mass does not really affect the distance traveled, it is not added into the equation.

The distances traveled using this equation are stated in part (a) of the explanation.