Answer:
The magnitude of total acceleration of the top of the racket is 402.5 m/s².
Step-by-step explanation:
Given:
Angular acceleration of racket (α) = 170 rad/s²
Angular speed of the racket (ω) = 18.0 rad/s
Distance between the top of racket and shoulder (r) = 1.10 m
Now, the magnitude of total acceleration is given as the square root of the sum of the squares of its radial and tangential components.
Radial component of acceleration is given as:
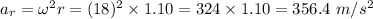
Tangential component of acceleration is given as:
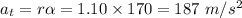
Now, magnitude of total acceleration is given as:
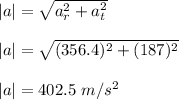
Therefore, the magnitude of total acceleration of the top of the racket is 402.5 m/s².