Question

A cylinder is being flattened so that its volume does not change. Find the rate of change of radius when r = 3 inches and h = 5 inches, if the height is decreasing at 0.6 in/sec. Hint: what is the rate of change of volume?

Answer 1

The radius is increasing at a rate 0.18 inches per second.

Explanation:

We are given the following in the question:

The volume of cylinder is constant.

`(dV)/(dt) = 0`

`(dh)/(dt) = -0.6\text{ inch per second}`

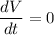
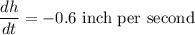
Instant radius= 3 inches

Instant height = 5 inches

Volume of cylinder =

`V = \pi r^2 h`

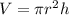
Rate of change of volume =

`(dV)/(dt) = \pi(2r(dr)/(dt)h + r^2(dh)/(dt))`

Putting all the values, we get,

`0 = (22)/(7)(2(3)(dr)/(dt)(5)+(3)^2(-0.6))\\\\30(dr)/(dt) = 9(0.6)\\\\(dr)/(dt) = (9* 0.6)/(30) = 0.18`

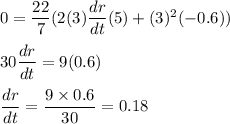
Thus, the radius is increasing at a rate 0.18 inches per second.