Question

Researchers have a sample size of 24 , and they are using the Student's t-distribution. What are the degrees of freedom and how would they notate this distribution?

Answer 1

For this case assuming that the random variable is X

`df = n-1`

And replacing n = 24 we got:

`df = 24-1=23`

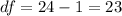
And we notate the distribution we got:
`X \sim t_(n-1)= t_(23)`

Explanation:

Previous concepts

The t distribution (Student’s t-distribution) is a "probability distribution that is used to estimate population parameters when the sample size is small (n<30) or when the population variance is unknown".

The shape of the t distribution is determined by its degrees of freedom and when the degrees of freedom increase the t distirbution becomes a normal distribution approximately.

The degrees of freedom represent "the number of independent observations in a set of data. For example if we estimate a mean score from a single sample, the number of independent observations would be equal to the sample size minus one."

Solution to the problem

For this case assuming that the random variable is X

`df = n-1`

And replacing n = 24 we got:

`df = 24-1=23`

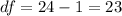
And we notate the distribution we got:
`X \sim t_(n-1)= t_(23)`