Answer:
Probability that fewer than 320 out of the n = 750 adults over 65 in the study suffer from one or more of the conditions under consideration is 0.0934 .
Explanation:
We are given that In the U.S., 45% of adults over 65 suffer from one or more of the conditions considered in the study. And we have to find the probability that fewer than 320 out of the n = 750 adults over 65 in the study suffer from one or more of the conditions under consideration.
Firstly, the above situation can be represented through binomial distribution, i.e.;

where, n = number of samples taken = 750
r = number of success
p = probability of success, i.e. 45%
Now, we can't calculate the required probability using binomial distribution because here n is very large, so we will convert this distribution into normal distribution using continuity correction.
So, Let X = No. of adults suffering from one or more of the conditions under consideration
Mean of X,
 =
=
 =
=
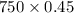 = 337.5
= 337.5
Standard deviation of X,
 =
=
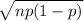 =
=
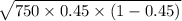 = 13.62
= 13.62
So, X ~ N(
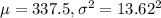 )
)
Now, the z score probability distribution is given by;
Z =
 ~ N(0,1)
~ N(0,1)
So, probability that fewer than 320 out of the n = 750 adults over 65 in the study suffer from one or more of the conditions under consideration is given by = P(X < 319.5) ---- using continuity correction
P(X < 319.5) = P(
 <
<
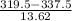 ) = P(Z < -1.32) = 1 - P(Z
) = P(Z < -1.32) = 1 - P(Z
 1.32)
1.32)
= 1 - 0.90658 = 0.0934
Therefore, required probability is 0.0934.