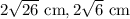Answer:
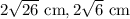
Explanation:
We are given the following in the question:
Two sides of triangle:
8 cm and
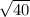 cm
cm
Let the third side be x cm.
Pythagoras theorem:
The sum of square of two sides of a right angled triangle is equal to the square of hypotenuse.
If the third side is hypotenuse, then by Pythagoras theorem:
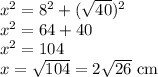
If the third side is not hypotenuse, then
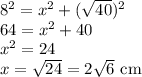
Thus, possible third side of triangle are