Question

A large power plant generates electricity at 12.0 kV. Its old transformer once converted the voltage to 385 kV. The secondary of this transformer is being replaced so that its output can be 500 kV for more efficient cross-country transmission on upgraded transmission lines.

(a) What is the ratio of turns in the new secondary compared with turns in the old secondary
(b) What is the ratio of new current output to old output (at 425 kV) for the same power?
(c) If the upgraded transmission lines have the same resistance, what is the ratio of new line power loss to old?

Answer 1

a) In the new transformer there are 42 turns in the secondary per turn in the primary, while in the old transformer there were 32 turns per turn in the primary.

b) The new output is 86% of the old output

c) The losses in the new line are 74% the losses in the old line.

Step-by-step explanation:

a) To relate the turns of primary and secondary to the ratio of voltage we have this expression:

`(n_1)/(n_2)=(V_1)/(V_2)`

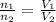
In the old transformer the ratio of voltages was:

`(n_1)/(n_2)=(V_1)/(V_2)=(12)/(385) =0.03117\\\\n_2=n_1/0.03117=32.1n_1`

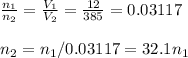
In the new transformer the ratio of voltages is:

`(n_1)/(n_2)=(V_1)/(V_2)=(12)/(500) =0.024\\\\n_2=n_1/0.24=41.7n_1`

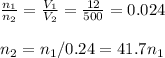
In the new transformer there are 42 turns in the secondary per turn in the primary, while in the old transformer there were 32 turns per turn in the primary.

b) The new current ratio is

`(V_1)/(V_2)=(I_2)/(I_1)=(12)/(500)= 0.024\\\\I_2=0.024I_1`

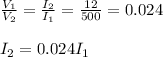
If the old current output was 425 kV, the ratio of current was:

`(V_1)/(V_2)=(I_2)/(I_1)=(12)/(425)= 0.028\\\\I_2=0.028I_1`

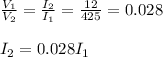
Then, the ratio of the new output over the old output is:

`(I_(2new))/(I_(2old)) =(0.024\cdot I_1)/(0.028\cdot I_1)= 0.86`

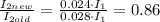
The new output is 86% of the old output (smaller output currents lower the losses on the transmission line).

c) The power loss is expressed as:

`P_L=I^2\cdot R`

Then, the ratio of losses is (R is constant for both power losses):

`(P_n)/(P_o) =(I_n^2R)/(I_o^2R) =((I_n)/(I_o) )^2=0.86^2=0.74`

The losses in the new line are 74% the losses in the old line.