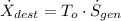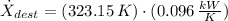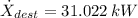Question

. Steam enters an adiabatic turbine at 4 MPa and 500 °C and exits at 50 kPa with a quality of 0.95. If the work output is 500 kW, assuming the surrounding temperature is 50 °C, find: a. The exit temperature of the steam (°C) b. The mass flow rate of air through the device (kg/s) c. The net rate of entropy generation for this process (kW/K)

Answer 1

a)
`T_(out) = 81.32\,^(\textdegree)C`, b)
`\dot m = 0.546\,(kg)/(s)`, c)
`\dot S_(gen) = 0.096\,(kW)/(K)`

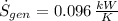
Step-by-step explanation:

a) After consulting a property table of saturated water, it is found that exit temperature of the steam is:

`T_(out) = 81.32\,^(\textdegree)C`

b) Rather, it is required to determine the mass flow rate of water through the turbine. Let assume that changes in kinetic and potential energy are negligible. The mass flow rate can be determined by applying the First Law of Thermodynamics:

`-\dot W_(out) +\dot m\cdot (h_(in)-h_(out))=0`

`\dot m = (\dot W_(out))/(h_(in)-h_(out))`

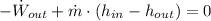
Properties at inlet and outlet are presented below:

Inlet - Superheated Vapor

`P = 4000\,kPa`

`T = 500^(\textdegree)C`

`h = 3446.0\,(kJ)/(kg)`

`s = 7.0922\,(kJ)/(kg\cdot K)`

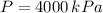
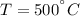
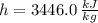
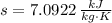
Outlet - Liquid-Vapor Mixture

`P = 50\,kPa`

`T = 81.32^(\textdegree)C`

`h = 2530.0\,(kJ)/(kg)`

`s = 7.26801\,(kJ)/(kg\cdot K)`

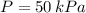
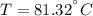
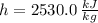
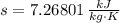
The mass flow rate is:

`\dot m = (500\,kW)/(3446.0\,(kJ)/(kg) - 2530.0\,(kJ)/(kg) )`

`\dot m = 0.546\,(kg)/(s)`

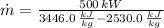
c) The net rate of entropy generation is constructed by using the Second Law of Thermodynamics:

`\dot m \cdot (s_(in)-s_(out)) + \dot S_(gen) = 0`

`\dot S_(gen) = \dot m \cdot (s_(out)-s_(in))`

`\dot S_(gen) = (0.546\,(kg)/(s))\cdot (7.26801\,(kJ)/(kg\cdot K)- 7.0922\,(kJ)/(kg\cdot K) )`

`\dot S_(gen) = 0.096\,(kW)/(K)`

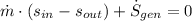
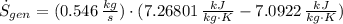
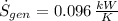
The surrounding temperature is only useful to estimate destroyed exergy, whose quantity is determined by the Gouy-Stodola Theorem:

`\dot X_(dest) = T_(o)\cdot \dot S_(gen)`

`\dot X_(dest) = (323.15\,K)\cdot (0.096\,(kW)/(K) )`

`\dot X_(dest) = 31.022\,kW`