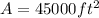Answer:
The length and width of plot is
 ,
,
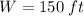
Largest area of the plot is
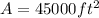
Explanation:
Assume width as x and length as y. Given that length of fencing is 600 feet and fencing is enclosed on 3 sides. So perimeter is given as,
Perimeter = width + length + width
Substituting the value,
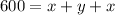
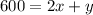 ….1
….1
Now area of fence of rectangular box is given as follows,
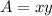 ….2
….2
Solving equation 1 for y, subtracting 2x from both sides,

Substituting the value in equation 2,
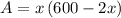
Simplifying
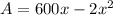
Rewriting,
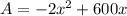
Above equation looks like quadratic equation
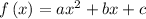 whose graph looks like parabola.
whose graph looks like parabola.
Comparing equation f(x) and A values of a, b and c are,
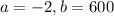 and
and
 .
.
Now maximum of
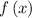 occurs at vertex.
occurs at vertex.
The x coordinate of the vertex is given as
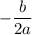
Substituting the values,
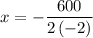
Simplifying,
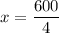
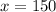
So width of plot is 150 feet.
Now to calculate value of length by using equation 1,
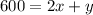
Substituting the values,
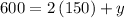
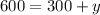
Subtracting 300 from both sides,
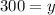
So length of plot is 300 ft.
The y coordinate of the vertex is given as
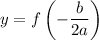 which also means,
which also means,

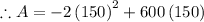
Simplifying,
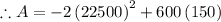
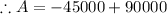
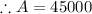
So, area of the plot will be